On May 9-10, ERC Nemo organizes a workshop on random measures, unimodularity and Palm theory. The workshop consists of four talks by Guenter Last (Karlsruhe Institute of Technology), Hermann Thorisson (University of Iceland), Roman Gambelin (Inria Paris) and Ali Khezeli (Inria Paris). It takes place both physically at INRIA Paris (2, rue Simone Iff, 75012) in salle Gilles Kahn and remotely.
To join the workshop remotely, you can connect to the following meeting: https://inria.webex.com/inria-
[inria.webex.com]
Meeting number: 2740 293 2122
Password: FbZtMhcG439
Invited speakers :
- Guenter Last (Karlsruhe Institute of Technology)
- Hermann Thorisson (University of Iceland)
- Roman Gambelin (INRIA Paris)
- Ali Khezeli (INRIA Paris)
Program:
Monday May 9:
- 10:30 AM – 11:30 AM: Roman Gambelin
- 2:00 PM – 3:00 PM: Guenter Last
Tuesday May 10:
- 11:00 AM – 12:00 AM: Ali Khezeli
- 3:30 PM – 4:30 PM: Hermann Thorisson
Titles and Abstracts:
Guenter Last (Karlsruhe Institute of Technology) – Tail processes, tail measures and Palm calculus
Tail processes and tail measures are important concepts in the theory of regularly varying (heavy tailed) time series. In this talk we will show that these concepts are intimately related to Palm theory of stationary random measures. To motivate the topic, we start with providing some background
on regularly varying time series. Then we shall introduce tail fields in an intrinsic way, namely as spectrally decomposable random fields
satisfying a certain space shift formula. The index set is allowed to be a general locally compact Hausdorff Abelian group. The field may take its values in an Euclidean space or even in an arbitrary measurable cone, equipped with a pseudo norm. We characterize mass-stationarity of the
exceedance random measure in terms of a suitable version of the classical Mecke equation. As a rule, the associated stationary measure is not finite.
We shall show that it is homogeneous, that is a tail measure. Finally we will establish a spectral representation of stationary tail measures.
The talk is based on the recent preprint https://arxiv.org/abs/2112.15380
Hermann Thorisson (University of Iceland) – Transportation of diffuse random measures on 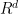 .
.
Abstract: Consider two jointly stationary and ergodic random measures with equal finite intensities, assuming one (the source) to be diffuse and the other (the destination) to be arbitrary. An allocation is a random mapping taking to
in a translation invariant way. We outline how to construct allocations transporting the diffuse source to the arbitrary destination, under the mild condition of existence of an ‘auxiliary’ simple point process which is needed only in the case when the destination is also diffuse. When that condition does not hold, we show by a counterexample that such an allocation need not exist. This is joint work with Guenter Last.
Roman Gambelin (INRIA Paris) – An integral characterization for a class of random probability measures
Abstract: Let be a random probability measure on a Polish space
which distribution is given
for some random
– valued
independent of
and a non-negative function
. Under a restrictive hypothesis on L, we will see that \zeta can be decomposed in law as
, where
is a random sequence such that
is a random allocation model ( “stick breaking process”) and
is independent. Moreover, the law of the joint sequence can be fully derived from L and the law of
. Under the additional assumption that the law of
be diffuse, we will see that a stronger equation characterizes Pitman-Yor processes with a diffuse base measure. This latter is an extension of a characterization of Dirichlet processes given by Guenter Last (2020). The arguments rely partly on classical stochastic geometry tools. In particular, we will give a Slivnyak-like theorem for Pitman-Yor processes !
with diffuse base measure interpreted as point processes on .
Based on a joint work with Bartlomiej Blaszczyszyn and Thomas Lehericy.
Ali Khezeli (INRIA Paris) – Unimodular Continuum Spaces
The scaling limit of various discrete models in probability theory are random pointed metric spaces that satisfy some kind of mass transport principle. With this motivating example, we will introduce the notion of unimodular continuum measured metic spaces. This notion is in some sense a common generalization of (the Palm version of) stationary point processes, (the Palm version of) stationary random measures, unimodular graphs and unimodular discrete spaces. Also, with the aim of studying scaling limits, various notions of `equivariant dimension’ are introduced. This is done based on a generalization of Palm theory to point processes on unimodular continuum spaces.

