 First eigenvalue and eigenvector bounds on a square (structured mesh, 1st order conforming finite elements) |
 First eigenvector bounds on an L-shaped domain (adaptively refined mesh, 1st and 2nd order discontinuous Galerkin elements) |
 Adaptively refined mesh, domain with a hole |
 Guaranteed inclusion of the first eigenvalue, domain with a hole |
 Eigenvalue and eigenvector bounds, 2-cluster on a square (left) and 3-cluster on an L-shaped domain (right), 1st order conforming finite elements |
Main results:
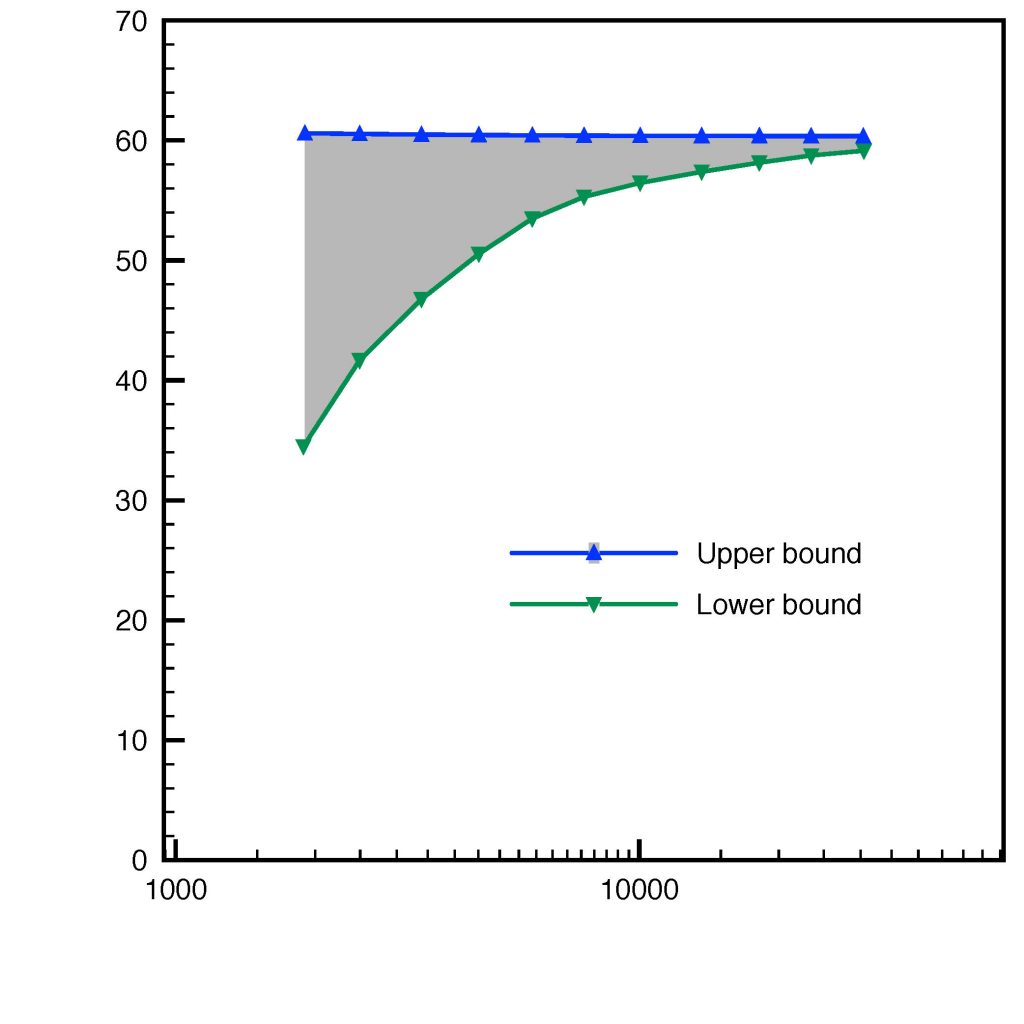
- guaranteed upper and lower bounds for an arbitrary simple eigenvalue, multiple eigenvalue, or a cluster of eigenvalues;
- guaranteed energy error bounds on the associated eigenvector(s);
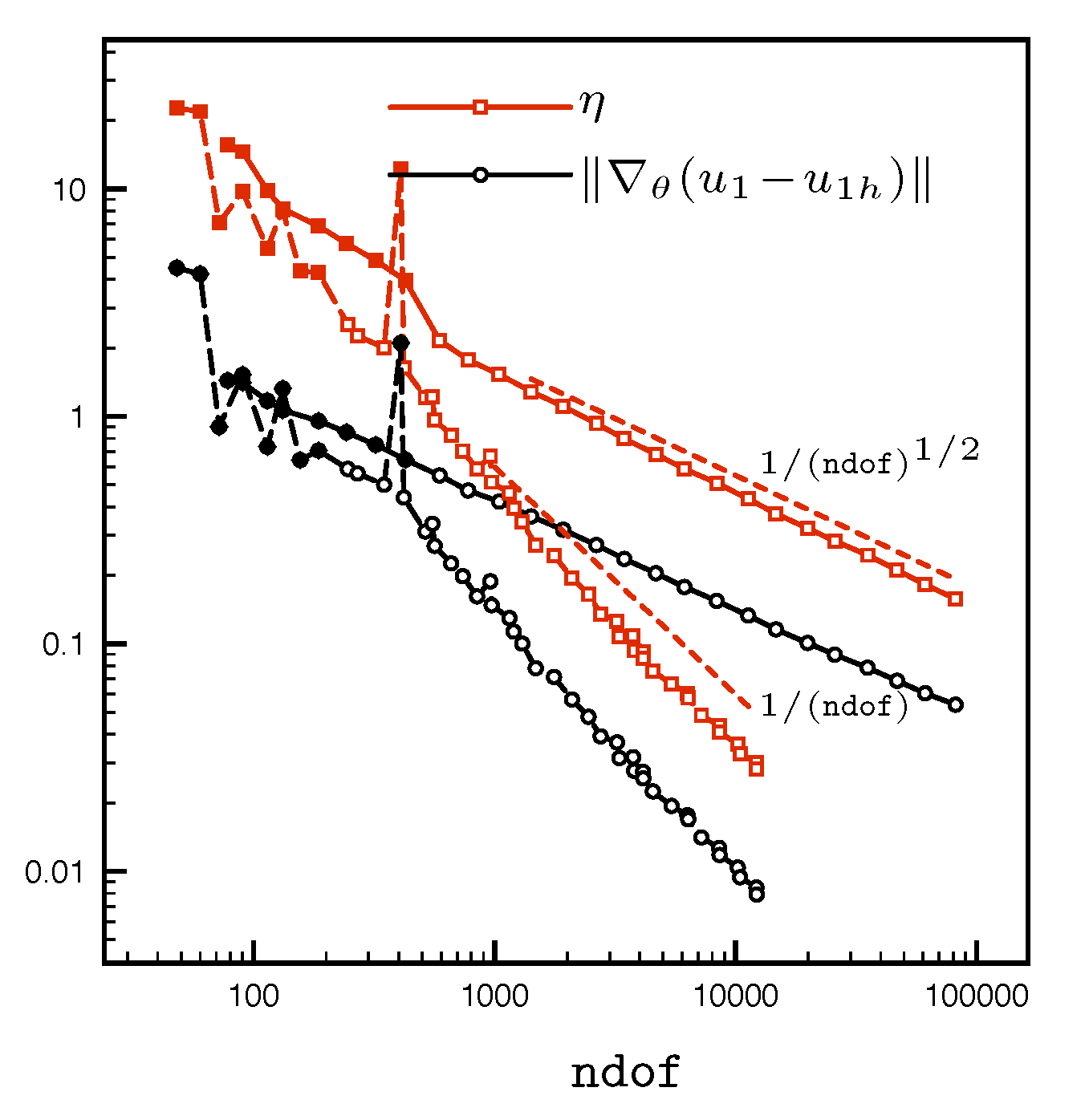
- efficiency (optimal convergence rate) and polynomial-degree robustness;
- multiplicative constant tending to one under an elliptic regularity assumption on the corresponding
source problem; - inexact algebraic solvers taken into account;
- unified framework covering basically any numerical method (conforming, nonconforming, discontinuous Galerkin, and mixed finite elements);
- abstract framework for any second-order self-adjoint elliptic linear operator with compact resolvent.
Details:
- with Eric Cancès, Geneviève Dusson, Yvon Maday, and Benjamin Stamm;
- conforming setting, simple eigenvalue: paper;
- unified framework, simple eigenvalue: paper (preprint) and presentation;
- conforming setting, multiple eigenvalues and clusters: paper (preprint), presentation.
