 Errors (left) and estimators (right), boundary layer |
 Error, error components, and estimators (left) and effectivity index (right), counter-example for no weights/submesh |
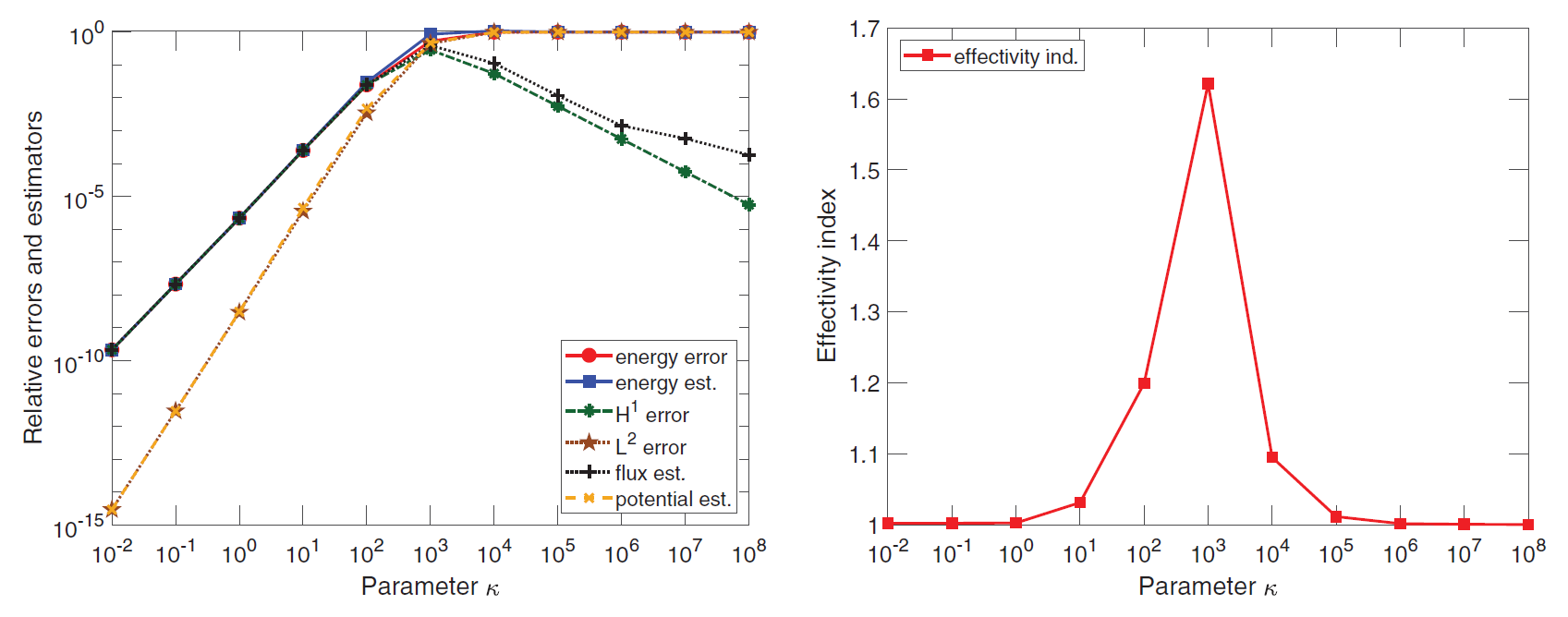
Main results:
- steady linear reaction–diffusion equation;
- arbitrary-order finite element approximation, arbitrary space dimension;
- a posteriori error estimates:
- guaranteed upper bound in the energy norm;
- local efficiency;
- robustness with respect to reaction dominance;
- inexpensive construction (weights; no submesh, no combination with residual estimates);
- counter-example where estimates with no weights/submesh/combination fail;
- explicit bounds for inverse inequality constants on a simplex.
Details: paper (preprint) with Iain Smears, presentation.
